The Checker Maven
Jump to navigationFind Both Solutions
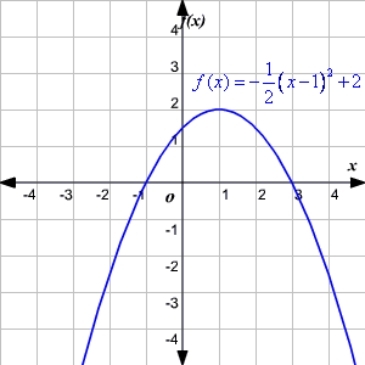
You probably learned in basic math classes that any quadratic equation has dual solutions, though they may not be unique, and when solving such equations, you were surely asked to find both solutions.
But as we've noted before, in checker problem competitions, "dual" solutions are frowned upon; a composition should have a but a single path to correctness. But with our speed problems, and with a mind to improving over the board visualization skills, sometimes a problem with a "dual" can be of value --- if you can find both solutions.
The following problem was sent to us by regular contributors Lloyd and Josh Gordon of Toronto, who developed it in conjunction with noted contemporary problemist Bill Salot. It has a dual solution.
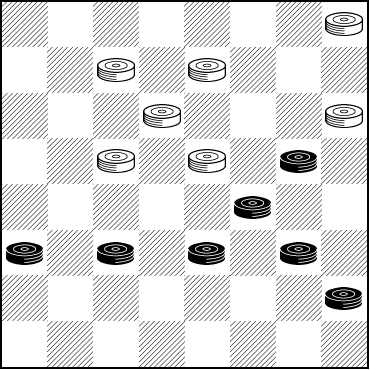
BLACK
Black to Play and Win
B:W18,19,21,23,26,27,29:B5,9,10,11,12,14,17.
Can you find both paths to victory? You'll get half credit for finding one of them, but full credit only if you work out both. The challenge is fair in that one solution is not simply a variant of the other.
Give this at least a "couple" of tries, and then click on Read More to see how you've done.![]()
Solution
The composer's solution is very simple and elegant:
12-16 19x12 10-15 26-22 17x26 27-24 15x22 Black Wins.
The computer solution seems a bit less attractive:
17-22 26x17 9-13 18x9 5x14 27-24 13x22 24-20 11-15 Black Wins.
Our thanks go out to Lloyd, Josh, and Bill.
You can email the Webmaster with comments on this article.
